Flat Tires Could Eventually Be A Thing Of The Past. Michelin Has Unveiled The Concept For A 3-D Printed,

There's an app for that

A “master list” of apps that are essential to student life.

Flashcards

Quizlet - Flashcard app that has pre downloaded flashcards and you can make your own flashcards. Available on multiple platforms

Duolingo - Language flashcard app

Flashcards+ - Another flashcard app

Math

Luna Calculator - calculator app ($2.99 on iOS)

Wolfram Alpha - online math solver

Symbollab - online math solver

Productivity & Planning

Forest - Grows a tree for every thirty minutes you are off your phone. If you touch your phone, the tree dies. ($0.99 on iOS)

FocusNow - Free version of Forest for iOS. Instead of trees, it grows fruits and vegetables. 

30/30 - Great time management app

Any.Do - Great app for creating to-do lists, organize tasks, syncs with other devices. You can also change the priorities on certain tasks. 

Studious - Great app on Android that reminds you when homework is due, when exams are and even silences your phone during class. 

StayFocused - A chrome extension that you can customize to limit the time on websites to stay productive

Self Control - Allows you to block your own access to distracting websites for a certain amount of time. Useful for people who get distracted on the computer (Also known as Cold Turkey for Windows).

My Study Life - Student planner app

myHomework Student Planner - another Student planner app

To-do List - extensive to do list, like an online bullet journal

Learning

Itunes U - For any apple device users, a app that gives you access to free educational courses 

TED - The whole catalog of TED talks at your fingertip. 

Wikipedia - Free encyclopedia that can be used for general references

Khan Academy - study videos (absolutely amazing app/website)

Music 

Coffitivity - coffee shop soundtrack

8tracks - great playlists, especially study playlists

Wake Up Calls

Alarmy - good alarm that will force you out of bed

Sleep Cycle - measures your sleep cycle

Math Alarm - alarm clock that forces you to do math to turn the alarm off, get’s your brain going 

Class Lectures and Note Taking

Dragon Dictation - takes notes for lectures (useful for classes where professors talk faster than you can type)

XMind - mind mapping tool

Notability - another app for recording lectures. ($2.99 on iOS)

These are just a few study apps that can be useful for studying. I will add more (or make a new list) later as I learn about them; feel free to message me to add some to this list. 

Hackaday Useful Tools Links

So I am an avid reader of Hackaday for a long time now and they have been putting out a lot of great introductions to tools and processes to get makers up to speed on the resources that are available.  This is just a splattering of links that I have found lately that you guys might be interested in.

DC Motors

Lessons in Small Scale Manufacturing

Grinding Gears: Figuring out gear ratios

Tools of the trade: Injection Molding

Are todays engineers worse?

How to nail a technical presentation

Tools of the trade: Vacuum Forming

The Art and Science of Bending Sheetmetal

A how-to of designing, fab, and assembly with structural framing systems (t slot)

Machine learning foundations

A machine shop in a box

How to: Cold resin casting

Join the GUI generation: Qtcreator

Do you guys have any other great resources that you’d like to share and/or are you enjoying this type of content?

Physical Science...In Space!

Each month, we highlight a different research topic on the International Space Station. In May, our focus is physical science.

The space station is a laboratory unlike any on Earth; on-board, we can control gravity as a variable and even remove it entirely from the equation. Removing gravity reveals fundamental aspects of physics hidden by force-dependent phenomena such as buoyancy-driven convection and sedimentation.

Gravity often masks or distorts subtle forces such as surface tension and diffusion; on space station, these forces have been harnessed for a wide variety of physical science applications (combustion, fluids, colloids, surface wetting, boiling, convection, materials processing, etc).

Other examples of observations in space include boiling in which bubbles do not rise, colloidal systems containing crystalline structures unlike any seen on Earth and spherical flames burning around fuel droplets. Also observed was a uniform dispersion of tin particles in a liquid melt, instead of rising to the top as would happen in Earth’s gravity. 

So what? By understanding the fundamentals of combustion and surface tension, we may make more efficient combustion engines; better portable medical diagnostics; stronger, lighter alloys; medicines with longer shelf-life, and buildings that are more resistant to earthquakes.

Findings from physical science research on station may improve the understanding of material properties. This information could potentially revolutionize development of new and improved products for use in everything from automobiles to airplanes to spacecraft.

For more information on space station research, follow @ISS_Research on Twitter!

Make sure to follow us on Tumblr for your regular dose of space: http://nasa.tumblr.com

But that’s not all it can do. Microsoft and NASA teamed up to “bring” you, yes you, to Mars.

Follow @the-future-now

These 17 Women Changed The Face Of Physics

Click through to read the rest.

A sponge can’t soak up mercury. (Video) Facebook | Instagram | Scary Story Website

IMPOSSIBLE! Right? You may have heard “the interior angles of a triangle always add up to 180 degrees”. This is not always true. Check out the second image, it shows a triangle with 3 right angles for a total of 270 degrees! 

It is true in flat Euclidean geometry (the geometry you probably learned in school) however. But there are so many other geometries out there! You may be thinking, are other geometries real though? A mathematician would argue they are just as real as the typical flat geometry you know and love (or hate). These alternative geometries can be practically useful too!

The images above show triangles in spherical geometry. Those aren’t triangles though! Oh but they are! A triangle is just a polygon enclosed by three lines. Looks like it fits the criteria. Wait but those aren’t lines, they are curved! Ah yes. I argue that these are, for all intents and purposes, just as good as lines. We need to ask: What is a line? A line is so basic to us we may not know how to describe it. I offer this definition: A line is the shortest path between 2 points. The 3 curves that make the triangle above are in fact the shortest paths from one vertex to the other on the surface of the sphere (they just so happen to be on circumferences of the sphere, which are often referred to as great circles). So it may be more useful to think of lines, in general, as length minimizing curves. In conclusion, we would consider the shape above to be a triangle as it is enclosed by 3 length minimizing curves on a surface.

Spherical geometry can be very useful; think about the Earth. To reduce travel time, airplanes would want to travel along great circles as they are the shortest paths from one place to another. Additionally, this type of thinking (rethinking straight lines as length minimizing curves) is central to Albert Einstein’s general theory of relativity.

read more at http://staffrm.io/@missnorledge/35H6cS1T52

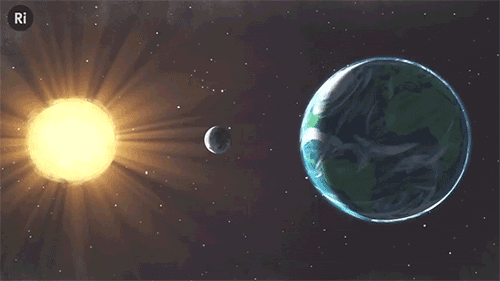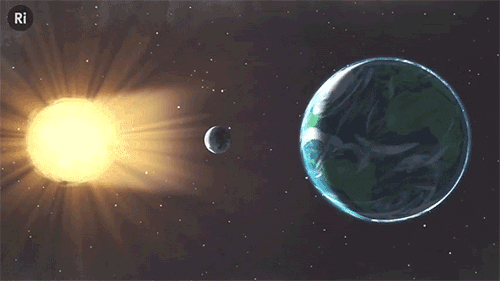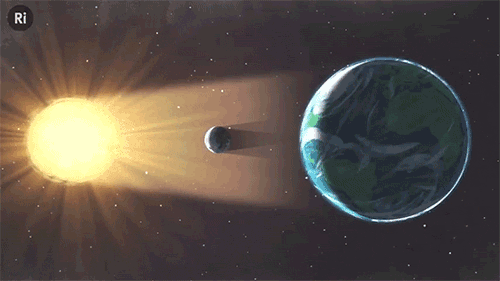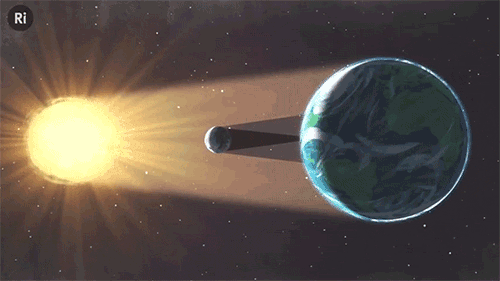
What have eclipses ever done for science? Quite a lot, actually!

The first measurement of the width of the Atlantic ocean in the 16th Century

When British settlers arrived in Virginia in the US, they weren’t sure how far across the globe they’d gone. They recorded the local time of a total eclipse of the moon - which is seen all across the night-time side of the planet. Their colleagues in London did the same, and when the travellers returned they could figure out the five hour time difference.

Edmond Halley discovered that the moon is moving away from the Earth

Halley realised you could back-calculate when previous eclipses would have occurred. But he noticed a mismatch between his predictions and the history books. The reason, he discovered, what that he was assuming the moon stayed the same distance from the Earth. It is actually getting further at about the rate your fingernails grow. And that means that one day (in a few million years, that is), the moon will be too far away to create any more total solar eclipses.

In 1919 a solar eclipse proved Einstein’s theory of relativity

Einstein’s theory predicted that the sun’s gravity should bend the light of nearby stars, meaning that in theory we should be able to see stars that are hidden just behind the sun. However, sunlight always blocks our view of these stars, and it was only during a solar eclipse that there was a short window to see if hidden stars were visible, as predicted. Astronomer Arthur Eddington travelled to West Africa and took photos that proved Einstein right.

Scientists still use solar eclipses today

It’s very hard to study the sun’s corona - a tenuous hot gas, which just one millionth of the light intensity of the sun. The shapes and lines of the corona show the nature of the sun’s magnetic field, and are only visible to study during an eclipse. NASA are also using this opportunity to help create the first thermal map of Mercury!

Want to know more? Watch our full video.

A portal to another universe ?

That my dear friends is a CT scan machine. Stripped off all the body parts, you can see clearly see what goes on inside.

A computerized tomography (CT) or computerized axial tomography (CAT) scan combines data from several X-rays to produce a detailed image of structures inside the body.

                                            CT scan of Brain

Pretty cool, don’t you think ?

Extras

Difference between MRI and CT scan

Why dont you spin the patient instead ? - Awesome reddit thread

Better quality gifs : here

Source Video: Micheal Jonnson

How did the Greeks know ?

Greeks had a strong geometric approach towards problems and as a result their methods are very intuitive.

In this post, we will look at the Method of exhaustion formulated by Archimedes that stands out as a milestone in the history of mathematics

Method of Exhaustion - Archimedes

                                                       Source

In order to find the bounds of pi, Archimedes came up with a remarkably elegant ‘algorithm’, which is as follows:

Lower bound

Inscribe a n-sided polygon in a circle —> Measure its perimeter(p) —> Measure its diameter(d) —> pi_min = p/d —-> Repeat with  n+1 sides.

Upper bound

Circumscribe a n-sided polygon in a circle —> Measure its perimeter(p) —> Measure its diameter(d) —> pi_max = p/d —-> Repeat with  n+1 sides.

And by following this procedure one could obtain the upper and lower bounds of pi !

Heres an animation made on geogebra for a circle of diameter 1. Watch how the lower and upper bounds vary.

Archimedes did this for a 96 sided polygon and found the value of pi  to be between 3.14103 and 3.1427. This is a good enough approximation for most of the calculations that we do even today!

Happy Holidays !